「百事數學/002」修訂間的差異

出自不錯學群
跳至導覽 跳至搜尋
 
（未顯示由 2 位使用者於中間所作的 37 次修訂）
行 1： 行 1：
 
[[分類:百事數學]]
 
[[分類:百事數學]]
−
=分數(一)=
+
=分數四則=
 
==前置準備==
 
==前置準備==
 
===一、材料：===
 
===一、材料：===
 +
<img src='http://jendo.org/uploadFiles/不錯學群/百事數學/分數/thumb/材料.jpg' width='200' height='*' />　<img src='http://jendo.org/uploadFiles/不錯學群//百事數學/分數/thumb/分數001.jpg' width='200' height='*' />
 
#A4 紙每人一叠
 
#A4 紙每人一叠
 
#筆
 
#筆
行 11： 行 12：
 
#麥克筆
 
#麥克筆
 
#可寫字的膠帶
 
#可寫字的膠帶
 +
#分數圖形產生器
  
 
===二、約定===
 
===二、約定===
行 18： 行 20：
 
#<math>\frac{1}{2}+\frac{1}{3}=</math>
 
#<math>\frac{1}{2}+\frac{1}{3}=</math>
 
#<math>\frac{2}{3}-\frac{1}{2}=</math>
 
#<math>\frac{2}{3}-\frac{1}{2}=</math>
−
#<math>3 \times 2</math>
+
#<math>3 \times 2=</math>
−
#<math></math>
+
#<math>3 \div 2=</math>
 
#<math></math>
 
#<math></math>
 +
參見[[zhtwwikipedia:使用說明:數學公式|數學公式使用說明]]
  
 
===四、復習===
 
===四、復習===
 +
[http://nice-learning.tw/uploadFiles/不錯學群/孫伯罕/xF3、243.htm http://nice-learning.tw/uploadFiles/不錯學群/孫伯罕/xF3、243.htm]
 +
 +
===五、造百事數學個人頁===
 +
#頁名為「百事數學/○○○」
 +
#每次上課標題為兩個等號，之下各段段標依序增加
 +
#在本次上課段落中增加兩個小段：
 +
#*找出五種非整數的日常生活中的量，如半個披薩。
 +
#*你認為日常生活中整數的量比較多？還是非整數的量比較多？你的理由是什麼？
  
 
==給加減乘除下「操作型定義」==
 
==給加減乘除下「操作型定義」==
 +
*加法：甲一堆，乙一堆，甲乙合起來，甲＋乙。注意：甲乙必須同單位才能相加。
 +
*減法：甲一堆，乙是甲其中的一小堆，從甲裡面把乙拿走，剩下的部分，甲－乙。注意：甲乙必須同單位才能相減。
 +
*乘法：
 +
*#甲一堆，同樣數量的甲有Ｎ堆，甲×Ｎ代表甲的Ｎ倍。<br/><br/>
 +
*#甲為寬，乙為高，甲×乙=面積：定義面積是 1×1 的幾倍。<br/><br/>
 +
*除法：
 +
*#甲(量)÷乙(量)：甲是乙的幾倍。<br/><br/>
 +
*#甲(量)÷乙(倍)：多少的乙倍會是甲？<br/><br/>
 +
*#距離÷時間=速率：定義新量速率<br/><br/>
 +
*#電壓÷電流=電阻：定義新量電阻<br/><br/>
 +
*#面積÷長=寬，面積÷寬=長
 +
 +
◉ 代表四則運算(＋ − × ÷)中的某一種運算。
 +
===一、交換律===
 +
甲◉乙 等於 乙◉甲
 +
#加法符合「交換律」，減法不符合「交換律」。
 +
#乘法符合「交換律」，除法不符合「交換律」。
 +
 +
===二、結合律===
 +
(甲◉乙)◉丙 等於 甲◉(乙◉丙)
 +
#加法符合「結合律」，減法不符合「結合律」。
 +
#乘法符合「結合律」，除法不符合「結合律」。
 +
 +
===三、分配律===
 +
<img src='https://upload.wikimedia.org/wikipedia/commons/thumb/6/69/Distributive_property_with_rectangles.svg/1024px-Distributive_property_with_rectangles.svg.png' width='640' height='165' />
 +
 +
例子：(2+3)×4=2×4+3×4
 +
<table style='border:none'><tr><th style='border:none'><table class=wikitable><tr><th style='background-color:#ccffaa'></th><th style='background-color:#ccffaa'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th></tr><tr><th style='background-color:#ccffaa'></th><th style='background-color:#ccffaa'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th></tr><tr><th style='background-color:#ccffaa'></th><th style='background-color:#ccffaa'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th></tr><tr><th style='background-color:#ccffaa'></th><th style='background-color:#ccffaa'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th></tr></table></th><td style='border:none;width:15%;'>＝</td><th style='border:none'><table class=wikitable><tr><th style='background-color:#ccffaa'></th><th style='background-color:#ccffaa'></th></tr><tr><th style='background-color:#ccffaa'></th><th style='background-color:#ccffaa'></th></tr><tr><th style='background-color:#ccffaa'></th><th style='background-color:#ccffaa'></th></tr><tr><th style='background-color:#ccffaa'></th><th style='background-color:#ccffaa'></th></tr></table></th><td style='border:none;width:15%;'>+</td><th style='border:none'><table class=wikitable><tr><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th></tr><tr><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th></tr><tr><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th></tr><tr><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th><th style='background-color:#aaaaff'></th></tr></table></th></tr></table>
  
 
==標示分數==
 
==標示分數==
 +
<img src='http://jendo.org/uploadFiles/不錯學群/百事數學/分數/thumb/分數002.jpg' width='200' height='*' />　<img src='http://jendo.org/uploadFiles/不錯學群/百事數學/分數/thumb/分數003.jpg' width='200' height='*' />　<img src='http://jendo.org/uploadFiles/不錯學群/百事數學/分數/thumb/分數006.jpg' width='200' height='*' /><br/><br/>
 +
 +
<img src='http://jendo.org/uploadFiles/不錯學群/百事數學/分數/thumb/分數009.jpg' width='200' height='*' />　<img src='http://jendo.org/uploadFiles/不錯學群/百事數學/分數/thumb/分數008.jpg' width='200' height='*' />　<img src='http://jendo.org/uploadFiles/不錯學群/百事數學/分數/thumb/分數005.jpg' width='200' height='*' /><br/><br/>
 +
 +
<img src='http://jendo.org/uploadFiles/不錯學群//百事數學/分數/thumb/分數004.jpg' width='200' height='*' />　<img src='http://jendo.org/uploadFiles/不錯學群/百事數學/分數/thumb/分數007.jpg' width='200' height='*' />　<img src='http://jendo.org/uploadFiles/不錯學群/百事數學/分數/thumb/分數012.jpg' width='200' height='*' /><br/><br/>
  
 
==擴分，約分==
 
==擴分，約分==
行 41： 行 85：
 
==比大小==
 
==比大小==
 
甲班吃 盒蛋糕，乙班吃了 盒蛋糕
 
甲班吃 盒蛋糕，乙班吃了 盒蛋糕
 +
 +
==分數相加==
 +
<math>\frac{1}{2}+\frac{1}{3}=\frac{5}{6}</math>
 +
 +
==分數相減==
 +
<math>\frac{2}{3}+\frac{1}{2}=\frac{1}{6}</math>
 +
 +
==分數相乘==
 +
#<math>4 \times 2=</math>
 +
#<math>4 \times \frac{1}{2}=</math>
 +
#<math>4 \times \frac{2}{3}=</math>
  
 
==除以分數==
 
==除以分數==
 
<videoflash>nZIHFgQP4rM</videoflash>
 
<videoflash>nZIHFgQP4rM</videoflash>
 +
 +
==畫圖做 html 檔==
 +
 +
==未來幾週學習路徑==
 +
#1 以上進位(百事數學/001)
 +
#1 以下分數(非整數)
 +
#1 以下十進位
 +
#1 科學記號
 +
#x 座標 y座標

於 2020年12月22日 (二) 14:02 的最新修訂

分數四則

前置準備

一、材料：

　

  1. A4 紙每人一叠
  2. 筆
  3. 尺
  4. 分數積木
  5. 色紙帶
  6. 剪刀
  7. 麥克筆
  8. 可寫字的膠帶
  9. 分數圖形產生器

二、約定

  1. 6 公分見方為 1
  2. 檔名規則：frac_x{1}{2}.svg，frac_y{2}{3}.svg，frac_x{1}{2}_y{2}{3}.svg

三、練習在 wiki 頁上寫分數

  1. [math]\frac{1}{2}+\frac{1}{3}=[/math]
  2. [math]\frac{2}{3}-\frac{1}{2}=[/math]
  3. [math]3 \times 2=[/math]
  4. [math]3 \div 2=[/math]
  5. [math][/math]

參見數學公式使用說明

四、復習

http://nice-learning.tw/uploadFiles/不錯學群/孫伯罕/xF3、243.htm

五、造百事數學個人頁

  1. 頁名為「百事數學/○○○」
  2. 每次上課標題為兩個等號，之下各段段標依序增加
  3. 在本次上課段落中增加兩個小段：
    • 找出五種非整數的日常生活中的量，如半個披薩。
    • 你認為日常生活中整數的量比較多？還是非整數的量比較多？你的理由是什麼？

給加減乘除下「操作型定義」

  • 加法：甲一堆，乙一堆，甲乙合起來，甲＋乙。注意：甲乙必須同單位才能相加。
  • 減法：甲一堆，乙是甲其中的一小堆，從甲裡面把乙拿走，剩下的部分，甲－乙。注意：甲乙必須同單位才能相減。
  • 乘法：
    1. 甲一堆，同樣數量的甲有Ｎ堆，甲×Ｎ代表甲的Ｎ倍。

    2. 甲為寬，乙為高，甲×乙=面積：定義面積是 1×1 的幾倍。

  • 除法：
    1. 甲(量)÷乙(量)：甲是乙的幾倍。

    2. 甲(量)÷乙(倍)：多少的乙倍會是甲？

    3. 距離÷時間=速率：定義新量速率

    4. 電壓÷電流=電阻：定義新量電阻

    5. 面積÷長=寬，面積÷寬=長

◉ 代表四則運算(＋ − × ÷)中的某一種運算。

一、交換律

甲◉乙 等於 乙◉甲

  1. 加法符合「交換律」，減法不符合「交換律」。
  2. 乘法符合「交換律」，除法不符合「交換律」。

二、結合律

(甲◉乙)◉丙 等於 甲◉(乙◉丙)

  1. 加法符合「結合律」，減法不符合「結合律」。
  2. 乘法符合「結合律」，除法不符合「結合律」。

三、分配律

例子：(2+3)×4=2×4+3×4

＝
+

標示分數

　　

　　

　　

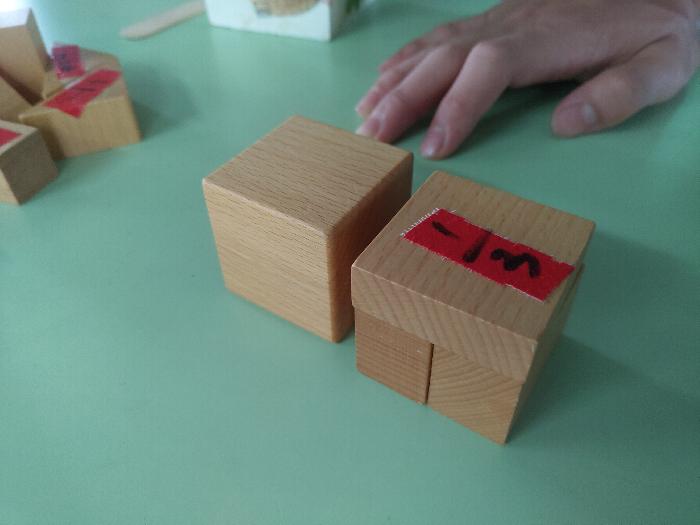
擴分，約分

一分數可以有多種表示法


百分比

  1. 10%
  2. 20%
  3. 25%
  4. 30%
  5. 50%

比大小

甲班吃 盒蛋糕，乙班吃了 盒蛋糕

分數相加

[math]\frac{1}{2}+\frac{1}{3}=\frac{5}{6}[/math]

分數相減

[math]\frac{2}{3}+\frac{1}{2}=\frac{1}{6}[/math]

分數相乘

  1. [math]4 \times 2=[/math]
  2. [math]4 \times \frac{1}{2}=[/math]
  3. [math]4 \times \frac{2}{3}=[/math]

除以分數

畫圖做 html 檔

未來幾週學習路徑

  1. 1 以上進位(百事數學/001)
  2. 1 以下分數(非整數)
  3. 1 以下十進位
  4. 1 科學記號
  5. x 座標 y座標
取自「http://nice-learning.tw/nlWiki/index.php?title=百事數學/002&oldid=3810」